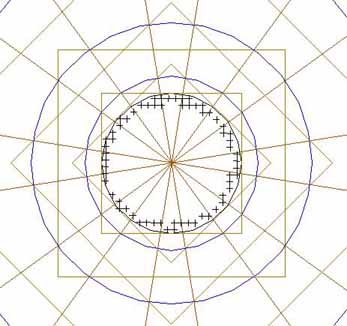
Figure 31: Architect, P. G Hunt's drawing of the centre post, greatly magnified. It is quite naturally contained within one of the PHI reducing squares. The code that the builders appear to have sought to encrypt into the centre post is the reciprocal of 1/2 PHI. The PHI squares reduce from the outer perimeter of the building until the 2nd to last one exhibits a diameter of, essentially, 4 feet (4.012196808 feet actual). If this were viewed as representing 4 feet exactly, then a direct PHI reduction on 4 feet would be 2.472136091 feet. In a pure PHI reduction from the outer perimeter the PHI square enclosing the centre post would be marginally larger at 2.479674133 feet. It is logical to assume that the ancient architect / mathematicians would have taken advantage of an excellent opportunity to place a series of dynamic codes into the centre post. This would have occurred in the following manner: The post would be fashioned to represent 2.472136091 feet of diameter, half of which is 1.236068045 feet (the reciprocal of 1/2 PHI...2 ÷ 1.6180339). Therefore the circumference of the post, in inches, would be 93.19755291" and this is exactly 2.88 Megalithic Yards of 32.360678 inches each. It is also 57.6 PHI inches (of 1.6180339" each) or 4.8 PHI feet (12 PHI inches or 19.41640681"). Remember these numbers...288...the diameter of the Aubrey circle, in feet, at Stonehenge and the basis of the Pyramid acre, which was 28800 square feet The length of the diagonal face of the Menkaure Pyramid was intended to be 288 feet to the apex; 576...the number 5.76 relates to the outer calibration of the Crosshouse itself, as its circumference value was 172.8 feet, rendering 1 degree of arc as 5.76 inches or .48 of a foot. The length up a diagonal side of the Great Pyramid was 576 feet to the top of the flat floor altar. Had the diagonal face lines from the bases of all 4 sides been allowed to converge to a common point above the Pyramid, then the vertical height to that point was intended to code 480 feet.
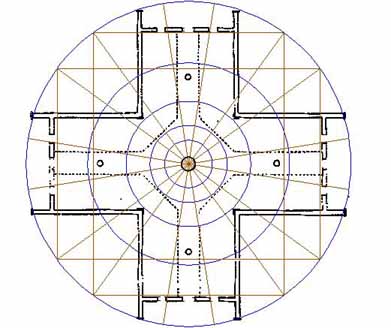
Figure 32: The Holy Rectangles, which were used as a simple means of achieving PHI relationships between circles and squares. Note how the rectangle lines delineate the internal limits of the building at the end of each wing.
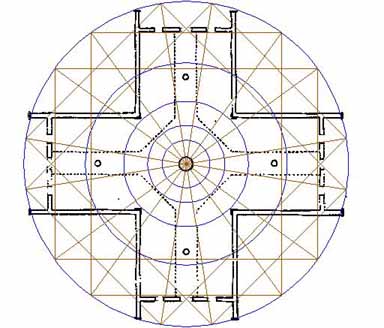
Figure 33: Offset Holy Rectangles, again showing a strong mathematical relationship to the design configuration of the Crosshouse. Drawing lines between the points where the Holy Rectangle vectors cross over the concentric PHI circles produce these rectangles.
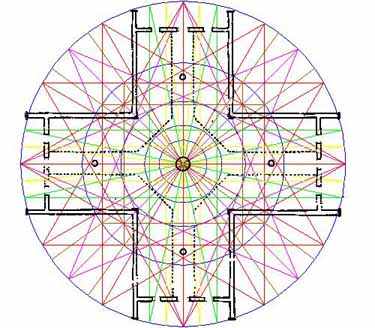
Figure 34: The "all in" geometry that would, generally, be used on a standing stone circle observatory to form the calculation matrix of 64 squares. All diagonal vectors will now be removed, except those relating to the 12-pointed star.
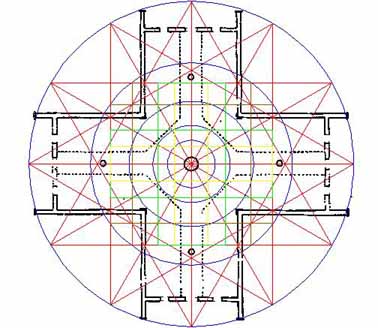
Figure 35: The calculation matrix of 64 squares, housed within the central square of the 12-pointed star. This configuration closely parallels some of the earliest zodiacs, which placed 3 zodiac constellations to each side of a square or quarter of the Earth. The design of the Crosshouse was clearly sufficient to code all major aspects of the "age-old" astronomical information, taught by successive, "Masters of the Craft". Initiates being schooled at Miringa te Kakara could learn all of the intricacies of the ancient arts and methods by reference to marked points on the building itself or by reference to positions of primary components of the Crosshouse structure. The 64-square matrix created from the overlay of star, cross and Holy Rectangle vectors is the origin of modern day chessboards, which are made up of 64 squares.

Figure 36: The 12-pointed star containing the 64 square, calculating matrix, swung 45 degrees. The centre post, along with the 4 secondary posts and internal corners of the outer porches, lock the 12-pointed star onto a precise grid or template, from which all other lines of the 64-square matrix can be determined. Note how each line is marked by either components of the building or intersections derived from lines running between components in conjunction with PHI circles.
It is certain that the Crosshouse of Miringa te Kakara complies to the ancient British Standard of measurement, in conjunction with traditional Northern Hemisphere geometric methodologies and copious usage of PHI values. The more individual codes, with their pedigree back to the Giza Plateau, can now be sought after and extracted from the cruciform building. The Crosshouse contains very clear codes in PI (3.1416) and further expresses those codes in accordance with British Standard foot values.
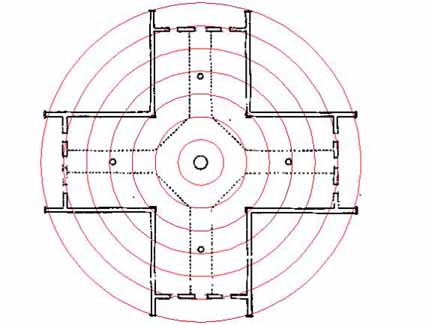
Figure 37: The circles starting from the centre and expanding outward, have a diameter of 1/4th PI in feet. Note how the 2nd circle relates to the walkway at the position where it turns from the diagonal onto the primary points of the compass. The 3rd circle brushes the "L" shaped, wrap-around corner mouldings and, as with the diagonal vectors of the 8 pointed star the edges of the moulding provide a perfect 3/4 PI code. The 4th circle has a diameter of 31.416 feet, rendering a perfect PI code. Note how it brushes the rear of the secondary posts indicating that they were purposely placed to code PI. The 6th circle swings to the end, exterior positions of the doorways and probably was meant to swing 3 inches beyond the outer wall line.
There might have been slight irregularities built into the lengths of the wings and these marginal differences might have been quite deliberate to incorporate additional codes, such as those related to PHI. The 7th circle swings to the end plates of the porches, leaving no doubt that a PI circle code was intended to brush inward of the plates. It would not be surprising to find post markers, beyond the building, which coded double PI...62.832 feet diameter...31.416 feet radius.
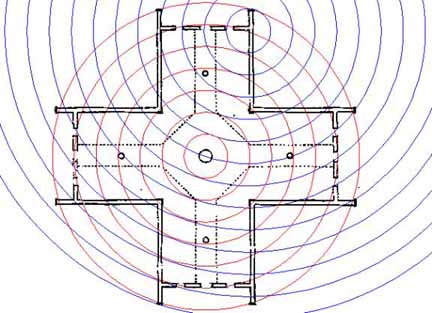
Figure 38: A second set of PI expanded circles with a diameter of 1/4th PI, coming from a corner position. Note the multiples of direct hits on primary parts of the building and how the two sets of circles meld with each other. This same effect, with only minor discrepancies, can be achieved from each internal corner at the ends of the wings. If all such PI relationships were added to this drawing, the sum total would be 111.
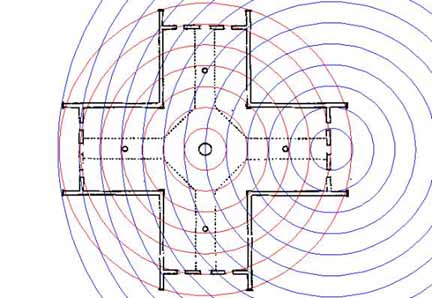
Figure 39: Yet more PI relationships, which can extend from each exterior door position and achieve excellent "hits" throughout the building. There are, potentially, 52 such relationships occurring from the exterior door fulcrums, taking into account that there are 4 doorways. If all were added to the tally, then the total, thus far, would be 163. It can be observed that the 2nd PI circle in this series designates the width of the wings, as well as how far the secondary posts are placed from the exterior doorway.
Without belabouring the point unnecessarily, it can be seen that several more points of the building can act as fulcrums to code PI increases in 1/4th PI increments (7.854 feet representing .7854...1/4th of 3.1416). It is not necessary to show all such relationships in drawn form as the existence of multiple PI codes within the confines of the building are adequately proven.
THE STONEHENGE PHI REDUCING CIRCLE CODES.

Figure 40: The relationships occurring in this picture show that whoever built the Crosshouse originally, encrypted the primary PHI circle codes of Stonehenge into the dimensions of the building. Whereas one of the PI circles, previously shown, had a diameter of 47.124 feet, one of the Stonehenge codes (at 1/10th the Stonehenge value) displays a close proximity value of 46.6 feet. The difference in the two values (47.124 or 46.6) is a little over 6 inches and if equally distributed to each side of the centre post, would mean an over-run beyond the porch wall line of 3 inches per side. Both circles (PI or PHI) appear to sweep to the outer line of the doorway entries. Its possible that two of the wings were slightly elongated to code the PI relationship and two were marginally shorter to code the Stonehenge circle of 466 feet (46.6 feet on the Crosshouse). The 466 feet diameter circle at Stonehenge is based upon a PHI increase of the Aubrey Circle (288 X PHI). Markers on the "Avenue" at Stonehenge indicate the diameter limits of the 466 feet circle.